Investigation in Acoustics of Wine Glasses

Introduction

Resonance is extremely important in engineering and structural design. It directly relates to the way buildings, bridges and other structures sway with disturbance. In the case of wine glasses used in the Glass Harmonica invented in 1761 by Benjamin Franklin, varying the amount of water contained within the glass will vary the resonant frequency of the glass.[1]

Get Help With Your Essay

If you need assistance with writing your essay, our professional essay writing service is here to help!
Find out more about our Essay Writing Service

The purpose of the task is to investigate how frequency varies as height of water increases in a range of wine glass diameters before making recommendations regarding the ideal height and diameter for a given frequency. Collection of data is not a simple task as the height of water must be measured under great scrutiny and the wave produced needs to be constant in order to accurately record frequency. However, alterations have been made to the design of the experimental setup and will account for error through these avenues. For example, volume of water will be increased in increments and the heights measured as increasing volume is easier than increasing height. Once the collection and processing of data has been completed, recommendations can be made about the use, manufacture and efficiency of the Glass Harmonica. Essentially, the aim of the investigation is to scrutinize the Glass Harmonica and make recommendations about other structures through extrapolation.

Background Theory

A.P. French’s Formula

While the Glass Harmonica is not the most commonly played instruments, the physics behind the way it works has been investigated a number of times.

A journal paper describing the resonance on wineglasses was written by the late A.P. French, a Ph. D. and former President of the American Association of Physics Teachers.[2] In the paper, French derived a general formula for how the frequency of a singing wineglass could vary with the volume of water in the glass.[3]

While French’s general formula was derived to describe the behaviour of ideal cylindrical glasses, it was found that any type of glass would approximately fit the formula. The formula is shown below:

Resonant Frequency

The main factor at play in the experimental investigation is resonant frequency. According to The Physics Classroom, “resonance is the tendency of a system to oscillate with greater amplitude at some frequencies than at others.”[4] The system’s resonant frequency is the frequency where the system demonstrates its relative maximum amplitude, that is, the system exhibits greatest oscillation.[5] Figure 1 illustrates the resonant frequency of a general system.

When its rim is rubbed by a moistened finger, the glass emits its resonant frequency. This is due to the crystals in the glass vibrating together which leads to one clear tone. As water is added to the glass, its resonant frequency changes.

Resonance is important on a bigger scale than just the use of the Glass Harmonica. It relates to the way structures and other man-made objects oscillate in the outside world. For example, the Takoma Narrows Suspension Bridge in Washington collapsed due to wind that was gusting at the exact resonant frequency of the bridge.[6] Furthermore, acoustic resonance is important for instrument builders, as many instruments use resonators, for example, strings on a guitar, the length of a tube and the tension on a drum membrane.

‘Slip-Stick Effect’

The slip-stick phenomenon is defined as “the spontaneous jerking motion that can occur while two objects are sliding over each other.”[7] The friction between two surfaces leads to a ‘stick’ effect. The ‘stick’ effect is due to the applied force not being great enough to overcome the friction. However, as the force applied becomes greater, one of the surfaces begins to ‘slip’. When the surface ‘slips’, the force applied increases the second surface’s velocity. As the velocity increases, the frictional force increases too, until the frictional force is greater than that of the force applied, leading to another ‘stick’. The process continues and is named the ‘slip-stick effect’.

The constant frictional jerking of the finger on the rim of the wine glass causes vibrations within the wall of the glass, leading to the oscillation of the glass and essentially, the tone produced.

How does the glass vibrate?

The glass begins to vibrate in a very special way when affected by the slip-stick phenomenon. When a moistened finger rubs along the glass, the rim begins oscillating into an elliptical shape due to its relatively elastic nature. Figure 2 portrays an exaggeration of the deformation of the rim of the glass.

The rim’s shape oscillates between the two elliptical shapes shown several hundred times per second, producing an audible tone.

Hypothesis

In context of the investigation to be undertaken, it is hypothesised that as height of water increases in each of the three glasses, the frequency produced by each of the glasses will fall. The glass that can contain the greatest volume of water will reduce the least over the course of the experiment. Additionally, both other glasses will have a greater rate of frequency decrease. Under test conditions, it is predicted that as the glasses get fuller, the frequency reduction will become greater as the stem of the glass supports the glass, hindering it from vibrating as much.

Correlation

Using French’s formula, a linear relationship can be established between the frequency produced and the height of water:

The value has been substituted into the equation as is built up of a number of constants representing the density of liquid, density of glass and glass thickness. Thus, plotting the following as and should present a linear relationship:

Graphing the above equation should present a value as gradient.

Ideal Graphs

Ideally, the graphs should be as depicted below:

The graph on the left depicts the reduction in frequency as height of water increases. The frequency slowly decreases in the first part before rapidly diminishing as height increases. The graph on the right has been manipulated using the raw data into a straight-line graph. Its gradient is the value.

Method

Clear the area and prepare the test glass and all other equipment used in experimentation. Place test glass flush on the desk before adopting silence in the room. Moisten index finger and begin softly rubbing the rim of the glass. Continue rubbing the rim of the glass until a standing wave appears. Begin recording sound in the room for a period of 10 seconds. If the standing wave is lost before the end of 10 seconds, stop the recording, delete the recording and repeat the procedure. If the standing wave continues, stop the recording at 10 seconds and stop rubbing the rim of the glass. Open the ANALYSE drop-down menu and select PLOT SPECTRUM. Trace along the graph until the peak is reached and record the frequency of the peak. Close the spectrum and delete the recording. Repeat 3 times. Measure out 20ml of water in a surgical syringe and add this liquid to the glass. Repeat the method outlined above.

The setup of the experiment is pictured below:

Results

The results of the experiment are tabulated below:

Raw Data

Analysis

Frequency Reduction (Hz)

Glass 1

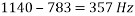
Glass 2

Glass 3

Linear Relationship Graphs

Glass 1

Glass 2

Glass 3

‘-Value’ for Different Glasses

Error Analysis

There are three forms of error in this experiment:

• Straight line error
• Measurement error
• Expected error

Straight Line Error

Measurement Error

Measurement error can be calculated using the smallest division of every piece of equipment used to measure values. These are presented below:

• Vernier: 0.01mm
• Audacity’s Frequency Spectrum: 0.5 Hz
• Syringe: Negligible as the volume increments are not factored into the French’s formula

Substituting various values into a rearranged version of French’s formula will find the various amounts of measurement error in each trial. The calculations are available below:

Formula

Glass 1

Therefore, measurement error is 0.52 Hz

Glass 2

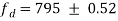
Therefore, measurement error is 0.52 Hz

Glass 3

Therefore, measurement error is 0.52 Hz

Expected Error

Expected error can be found by substituting the value for various glasses into the manipulated formula used for the measurement error. The result of graphing this is the expected frequency decrease curve. The graphs are presented below:

Glass 1

Glass 2

Glass 3

Average Difference Throughout the Duration of the Experiment

Maximum Difference

Discussion

Interpretation of Results

According to the results, the previously formulated hypothesis was proven correct. This is true since the frequency produced by each of the glasses fell as the height of water in each of the three glasses increased. Furthermore, Glass 2, which has the greatest capacity, also followed suit as it had the least frequency reduction. Moreover, stem of the glass acted as an excellent support for each of the glasses, ensuring that the raw graphed data followed a similar pattern to that expected. Another noteworthy trend was that the taller glass with the smallest capacity and radius had the greatest reduction in frequency. On the other hand, the shortest glass has the most stable and predictable decrease.

Following French’s formula, justification can be made as to why the values didn’t increase as height of glass increased. The values of each of the glasses is made up of the following:

Where the only variable factors between glasses are , radius of the glass and , thickness of the glass at water level. Thus, as increases, as with Glass 2, the value increases too. Naturally, as decreases, as with Glass 3, the value increases. Glass 3 had a higher value than Glass 1 simply due to the thin nature of the glass. Furthermore, Glass 2 had the highest value due to its large radius and almost spherical shape.

While it was not a part of this experimental investigation at all, it must be noted that the glass with the greatest value produced the loudest sound, that is, the wave with the greatest amplitude. An interesting observation can be made through linking the nature of the glass, the value and the amplitude of the sound wave produced. As the glass becomes thinner and rounder, the value increases, which in turn, leads to a louder sound being produced.

While the results obtained from the experiment are as were hypothesized, the outcome for the overall investigation is not as straightforward. The varied frequency decrease in the three glasses indicates which would be the most efficient in a Glass Harmonica with limited glasses. The dissimilarity also shows which glass would be able to play a specific small range more precisely than others. There are distinct advantages/disadvantages regarding high/low frequency reduction. The main advantage of the greater variation in frequency is that one can play a whole range of notes with only a few of the same type of glass. Additionally, the primary disadvantage of a great frequency decrease is that subtle changes in frequency cannot be easily made. A method of eliminating this disadvantage is simply using glasses that have a slower frequency reduction, such as Glass 2. However, this has its own advantages and disadvantages. The key advantage is that more specific notes in a small range can be played. Nevertheless, a disadvantage of this is that a large number of glasses need to be used, to play each specific note.

In the real world, when a Glass Harmonica is used, a whole range of glasses are used due to the fact that more precise notes can be played in a while range of frequencies. This is what makes these instruments so expensive. Usually, the higher notes are played using thinner glasses and lower, deeper notes are played using rounder, wider glasses.

Comparison with Expected Results

The results obtained from conducting the experimental investigation are slightly deviant from those expected. It was expected that the values of the various glasses would be ordered the same way as the retention of frequency, and in the following order, from greatest to smallest frequency retention:

The results obtained are divergent from these and follow the pattern as shown below:

However, when comparing the data collected to the expected data, there is a trend on all the graphs as they all begin almost exactly on par with the expected results. Glass 3 had the greatest amount of difference from the expected graph. On average, every frequency measured was 32.25 Hz above or below the value it should have been at. In addition, Glass 2 began on par wuth the expected curve before reducing frequency slightly slower than expected.

Nevertheless, the graphs were most consistent in both the beginning and end of each glass. As visible on the all three of the “difference in frequency” graphs, the true data began and ended almost exactly equal to the expected values.

While results obtained were fairly accurate, the maximum difference between the expected values and true data in the three glasses was 68.04 Hz.

Mistakes, Uncertainties, Errors

While the investigation undertaken does not blatantly show evidence of any significant mistakes/errors, there are certainly a number of anomalies. For example, Glass 3 had a greater value than Glass 1 even though it has a minute radius. The values of the various glasses differ by only a small amount and the reduction of frequency differ by a fairly large amount. Both these must be duly noted.

When analysing the raw data, there is a distinct anomalistic middle of all 3 of them. This is a clear indication of a large error caused by either measuring incorrectly each glass was further tested or simple inconsistencies in the peaks of Audacity’s frequency spectrum. Regardless, this error in all 3 experiments caused a deviance from the trendline. Unfortunately, it was not possible to avoid the influence of this error as values had to be calculated using those sections of data.

There are a number of errors, caused by the method, which could have influenced the results. Firstly, when measuring the values of height of water and height of glass through the Vernier, there existed a chance of parallax error as the readings may be slightly deviant from the true values. Secondly, increasing volume of water instead of height of water for ease of measurement may not have had the correct effect and it may have been easier to simply measure heights in standard increments. Lastly, the standing wave may have broken at points, leading to the peaks of the frequency spectrum having an effect on the validity of the results, for example, the raw data and it’s difference to the expected data wou